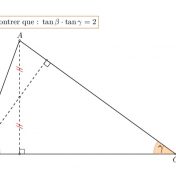
Dans un parallélogramme, la somme des carrés des diagonales égale la somme des carrés des côtés. Si les longueurs des côtés sont notées \(L\) et \(\ell\) et les longueurs des diagonales sont \(D\) et \(d\) alors il s’agit de montrer l’égalité $$D^2+d^2 = 2\ell^2+2L^2.$$ Cela devient simple si l’on considère que notre parallélogramme a pour sommets \(0\), \(z\), \(z’\) et… Read more »
Évariste Galois (1811-1832) était un mathématicien français qui demeure une figure légendaire dans l’histoire des mathématiques. Sa vie courte mais passionnée est marquée par son génie précoce et ses contributions révolutionnaires à l’algèbre abstraite. L’histoire de Galois révèle une anecdote remarquable de sa jeunesse lorsqu’il tenta d’intégrer l’École Polytechnique française. Malheureusement, son oubli d’un simple curriculum vitae réclamé pour l’inscription… Read more »
Calculer \(\lim\limits_{x \rightarrow 0} \frac{1-\cos (4 x)}{\sin ^{2}(7 x)}\) Source : calculus – Mathematics Stack Exchange
\(\frac{20 ! \: \cdot \: 7 !}{6 ! \: \cdot \: 21 !}=\frac{207}{621}=\frac13\) \(\frac{175 ! \: \cdot \: 56 !}{55 ! \: \cdot \: 176 !}=\frac{17556}{55176}=\frac{7}{12}\) \(\frac{1500 ! \: \cdot \: 475 !}{474 ! \: \cdot \: 1501 !}=\frac{1500475}{4741501}=\frac{25}{79}\) \(\frac{29600 !\: \cdot \: 9361 !}{9360 ! \: \cdot \: 29601 !}=\frac{296009361}{936029601}=\frac{37}{117}=0,3162393162 \cdots\) Lien instagram
\(f(\frac12)\) sachant que \[\ f(x)=\sum_{n=0}^{\infty}\left(2 n+n^{2}\right) x^{n}\]
Un outil indispensable pour générer le code latex de n’importe quel formule mathématique affichée à l’écran. Source : Mathpix Snip
Source : Les formules liées au crédit
Il suffit de trouver \(\alpha\) en radian et sous forme fractionnaire. \documentclass[border=5mm]{standalone} \usepackage{tkz-euclide} \usetkzobj{all} \begin{document} \begin{tikzpicture} \tkzDefPoint(0,0){O} \foreach[count=\i] \ANG in {22.5,67.5,112.5,157.5,202.5,247.5,292.5,337.5} { \tkzDefPoint({5*cos(\ANG*pi/180)},{5*sin(\ANG*pi/180)}){P\i} } \tkzDrawPoints[color=red,fill=white,size=7](P1,P2,P3,P4,P5,P6,P7,P8) \tkzInterLL(P1,P8)(P6,P7) \tkzGetPoint{I} \tkzDefSquare(P1,I) \tkzGetPoints{C}{D} \tkzDrawPolygon[color=black,ultra thick](P1,D,C,I) \tkzDrawPolygon[color=magenta, ultra thick](P7,P8,C) \tkzDrawPolygon[color=red,ultra thick](P1,P2,P3,P4,P5,P6,P7,P8) \tkzMarkAngle[fill=magenta!35,size=2.8cm,opacity=.5](P8,C,P7) \tkzLabelAngle[pos = 2.3](P8,C,P7){\color{black}{\mbox{\Large$\alpha$}}} \end{tikzpicture} \end{document}
Code source latex \documentclass[border=5mm]{standalone} \usepackage{tkz-euclide} \usetkzobj{all} \begin{document} \begin{tikzpicture} \tkzSetUpPoint[shape = circle,color = black,size = 5,fill = white] \tkzInit[xmin=0,ymax=6] \tkzClip[space=.5] \tkzDefPoint(0,0){B}\tkzDefPoint(-1,0){O} \tkzDefPoint(70:5.5){A} \tkzDefPointBy[projection= onto O–B](A) \tkzGetPoint{H} \tkzDefMidPoint(A,H) \tkzGetPoint{H’} \tkzDefLine[orthogonal=through A](B,H’) \tkzInterLL(A,tkzPointResult)(B,H) \tkzGetPoint{C} \tkzDefPointBy[projection= onto A–C](B) \tkzGetPoint{H »} \tkzLabelPoints[above](A) \tkzLabelPoints[below](B,C) \tkzDrawSegment[style=dashed](A,H) \tkzDrawSegment[style=dashed](B,H ») \tkzMarkSegments[mark=s||,color=red](A,H’ H’,H) \tkzDrawPolygon(A,B,C) \tkzMarkRightAngle(A,H,C) \tkzMarkRightAngle(B,H »,C) \tkzDrawPoints(A,B,C,H,H’,H ») \tkzMarkAngle[fill= yellow,size=1cm,opacity=.3](C,B,A) \tkzMarkAngle[fill= orange,size=1cm,opacity=.3](A,C,B) \tkzLabelAngle[pos = .7](C,B,A){\color{black}{\mbox{\Large$\beta$}}} \tkzLabelAngle[pos = .7](A,C,B){\color{black}{\mbox{\Large$\gamma$}}} \tkzText[draw=brown](3,6){Montrer que : $\tan \beta… Read more »
On montre que $$\int \:\tan ^n\left(x\right)\; \mathrm{d}x=\frac{\tan ^{n-1}\left(x\right)}{n-1}-\int \tan ^{n-2}\left(x\right)\; \mathrm{d}x$$ Etapes : \begin{align*} \int \:\tan ^n\left(x\right)\; \mathrm{d}x &= \int \tan ^{n-2}\left(x\right) \cdot \tan ^{2}\left(x\right)\; \mathrm{d}x \\ &= \int \tan ^{n-2}\left(x\right) \cdot \left(\frac{1}{\cos^2x}-1\right)\; \mathrm{d}x \\ &= \int \frac{\tan^{n-2}(x)}{\cos^2(x)} \; \mathrm{d}x \; – \int\tan^{n-2}(x)\; \mathrm{d}x \\ \end{align*} On pose et Par substitution, le premier terme du résultat précédent devient : \begin{align*}… Read more »